Finding relationships through mathematics

Advances in the tools researchers have at their disposal for exploring nature’s inner workings have been critical for continued scientific progress. Though this now often comes in the form of new sensors, processors, and measurement techniques, breakthroughs are still being made in one of the oldest tools used nearly universally throughout the sciences: mathematics.

Built on principles established over centuries, mathematics provides a means to represent and interpret various phenomena with formulas and numbers. But the exploration of various functions that weave numbers together in unique ways and relationships between numbers themselves has raised questions that have remained unsolved for generations—the answers to which could unlock new discoveries.

Masanobu Kaneko, professor at Kyushu University’s Faculty of Mathematics, has been searching for such answers related to multiple zeta values, numbers produced by a distinct formula for summing and multiplying integers out to infinity. Though some properties of these values were pondered by the great mathematician Leonhard Euler nearly 250 years ago, they have seen a surge in interest in recent years.

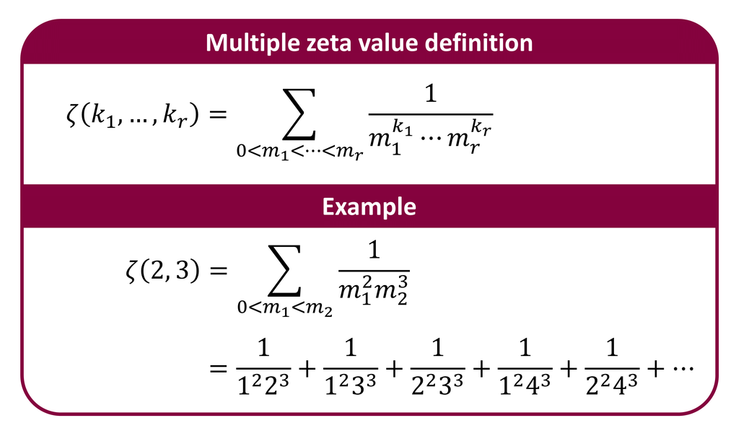
“Discovery of multiple zeta values in fields such as knot theory and theoretical physics played a big part sparking modern research into them,” says Kaneko.

“One place they turn up is Feynman diagrams, which describe the interactions of elementary particles—the most basic building blocks of matter. As such, I can’t help but wonder if multiple zeta values might possibly one day lead to prediction of a new type of particle.”

Kaneko’s fascination with the science of numbers began when he was a child. Initially drawn to astronomy, he would try to calculate the movement and orbits of planets and comets. When he realized in elementary school that this required high-level math, he began what would become his lifelong study of mathematics.

“I found mathematics compelling from the start. Beauty can be found throughout mathematics on many different levels,” Kaneko observes.

“For example, if you alternatively subtract and add the inverse of odd numbers up to infinity—one, minus one third, plus one fifth, minus one seventh, and so on—you get pi over four. Why does pi show up here? This hints at a beautiful, deeper connection between numbers, and proving why these connections exist can unlock new understanding.”

How Kaneko arrived at the subject of multiple zeta values is its own story of unexpected connections involving not just numbers but also people. Chance meetings and discussions with various colleagues and acquaintances about an idea that sprung from musings on a problem in a textbook by his previous professor eventually led him to pursue multiple zeta values, years after not giving them further consideration after first hearing about them at a seminar by a future collaborator.

Beyond just the present, mathematics also connects people across time as they work on the same problems centuries apart. But unlike the mathematicians of old, Kaneko is applying tools to his studies that have only become available recently.

“I often use computers to calculate numbers and look for new patterns. Experimentally manipulating numbers this way can help lead to new discoveries,” explains Kaneko.

Over his career spanning more than three decades, he has made several unexpected and exciting discoveries. One that stands out in particular for him is the surprising reproduction of a series of functions called modular forms when considering some particular parametrizations of certain elliptic curves.

“Very simply put, it was like finding out that a certain sentence is exactly the same despite using two completely different languages to describe something,” says Kaneko. “We still don’t know why this happens, but there must be a deeper relationship that no one has found yet.”

And it is these deep relationships—among numbers, functions, nature, and people—that underscore the beauty of mathematics for Kaneko.